مخطط الموضوع

• عام
  

  عام

  • Annonces منتدى
• Contact Form:
  

  Contact Form:

  
  

  Institute : Science and Technology

  
  

  Department: Common Core Department of Science and Technology.

  
  

  Target public: First year engineering (civil engineering, mechanical engineering, process engineering)

  
  

  Fields of Specialization: Mathematics

  
  

  Course title: Analysis 2

  
  

  Credit: 6

  
  

  Coefficient: 3

  
  

  Duration: 12 weeks

  
  

  Weekly timetable: 1h 30 min of course and 3 hours for tutorial group

  
  

  Place of teaching: Lecture hall 3 classrooms  9+10+11

  
  

  Teacher: Hocine RANDJI

  
  

  Contact: randji.h@centre-univ-mila.dz

                   Randji.Hocine@yahoo.com

  
  

  Evaluation methods: A final exam , short tests.

  
  

                                          The final evaluation is through
  
  

                                        • A written in-person exam at the end of the semester, covering all topics discussed in lectures
                                           as well as exercises from tutorial. This exam contributes to 60% of the final grade.
                                        • Continuous assessment during tutorial sessions, contributing to 40% of the final grade, divided
                                           into 14 points for short exams after each chapter and 6 points for presence and participation
                                           in each session.
                                            The passing grade for this course is equal to or greater than 10 out of 20
  
  
• Objectives of the teaching
  

  Objectives of the teaching

  Objectives of the teaching:
  

  
  

  
  

  1. Remembering:

  • Recall the fundamental concepts of integration and differentiation.

  • Remember key definitions related to infinitesimals, Taylor series, and differential equations.

  
  

  2. Understanding:

  • Understand the process of integrating functions and the concept of antiderivatives.

  • Grasp the significance of infinitesimals and their role in calculus.

  • Comprehend the Taylor series expansion of a function and its applications in function

  approximation.

  • Understand the basic terminology and concepts related to differential equations, such as

  order and linearity.

  
  

  3. Applying:

  • Apply different methods for integrating functions to solve problems.

  • Apply Taylor series expansions to approximate functions and evaluate their accuracy.

  • Apply techniques such as substitution and integration by parts to solve differential equations.

  
  

  4. Analyzing:

  • Analyze the behavior of functions near specific points and evaluate the accuracy of

  polynomial approximations.

  • Analyze and compare different orders of infinitesimals.

  • Analyze and classify differential equations based on their characteristics and types.

  
  

  5. Evaluating:

  • Evaluate the effectiveness of Taylor series approximations in solving real-world problems.

  • Evaluate the solutions obtained for differential equations and assess their correctness.

  
  

  6. Creating:

  • Develop computational algorithms to generate Taylor series expansions and solve differ-ential equations.

  • Create and implement Python scripts to enhance understanding and practical application of theoretical concepts
• Pre-requisites
  

  Pre-requisites

  

  Basic mathematics concepts (differential equations, integrals, systems of equations, ...)
  

  In case of not mastering the prior knowledge, you can refer, for example, to: 

  https://eddirasa.com/ens-sec/3as/maths/
  

  • Pre-test activity اختبار
• Content
  

  Content

  Chapter 1: Limited Developments (4 weeks) 

  The main topics in this chapter are:

  1. Comparison relations

  2. Limited developments in the vicinity of zero

  (a) Definitions of a limited development and Taylor-Lagrange theorem

  (b) Usual limited development

  (c) Operations on limited developments

  3. Limited developments in the vicinity of a point, in the vicinity of infinity and generalized

  limited developments.

  4. Applications of limited developments (calculation of limits, equations of tangent and

  asymptote)

  Chapter 2: Calculation of Primitives (5 weeks)

  The main topics in this chapter are:

  1. Definitions and properties (primitive, integral, and definite integral)

  2. Integration methods

  (a) Integration by parts

  (b) Integration by change of variable

  3. Integration of a rational fraction

  4. Integration of a rational fraction in sin and cos

  5. Integration of a rational fraction in exponential

  6. Integration of a rational in sin(h) or cos(h).

  Chapter 3: Differential Equations (3 weeks)

  The main topics in this chapter are:

  1. Definitions

  2. First-order differential equations.

  (a) Separable variable differential equations.

  (b) Linear differential equations.

  (c) Bernoulli differential equations.

  (d) Homogeneous differential equations with respect to x and y :

  3. Second-order linear differential equations with constant coefficients

  • Mind map ملف
  • Content صفحة
• Availability of the Teacher
  

  Availability of the Teacher

  
  

  
  

  I am available in the Teachers' Room, located on the first floor of the Science and Technology Building, every Monday and Wednesday from 10 AM to 12 PM. 
  

  • Wiki ويكي
  • Chat with your teacher in real time محادثة
• Chapter 01: Limited Developments
  

  Chapter 01: Limited Developments

  

  Specific objetives:
  

  • Understand and Apply Key Concepts: Grasp fundamental concepts related to infinitesimals and Taylor series, including definitions, theorems, and their applications. Develop the ability to identify and compare different orders of infinitesimals, and apply Taylor's formula to approximate functions.

  • Analyze and Solve Problems: Engage in problem-solving exercises that involve comparing infinitesimals, determining their equivalence, and using Taylor series for function approximation. Analyze the behavior of functions near specific points and evaluate the accuracy of polynomial approximations. 
  • Utilize Computational Tools: Implement computational techniques, such as using Python to generate Taylor series expansions, to enhance understanding and practical application of theoretical concepts. This includes creating, verifying, and applying series expansions

  • Exchange between Students منتدى
  • Chapter 01 ملف
  • Chapter 01 سكورم
  • Chapter 01 ملف
  • Tutorial exercises set 1 ملف
  • Test1 المهمة
• Chapter 02: Calculation of Primitives
  

  Chapter 02: Calculation of Primitives

  

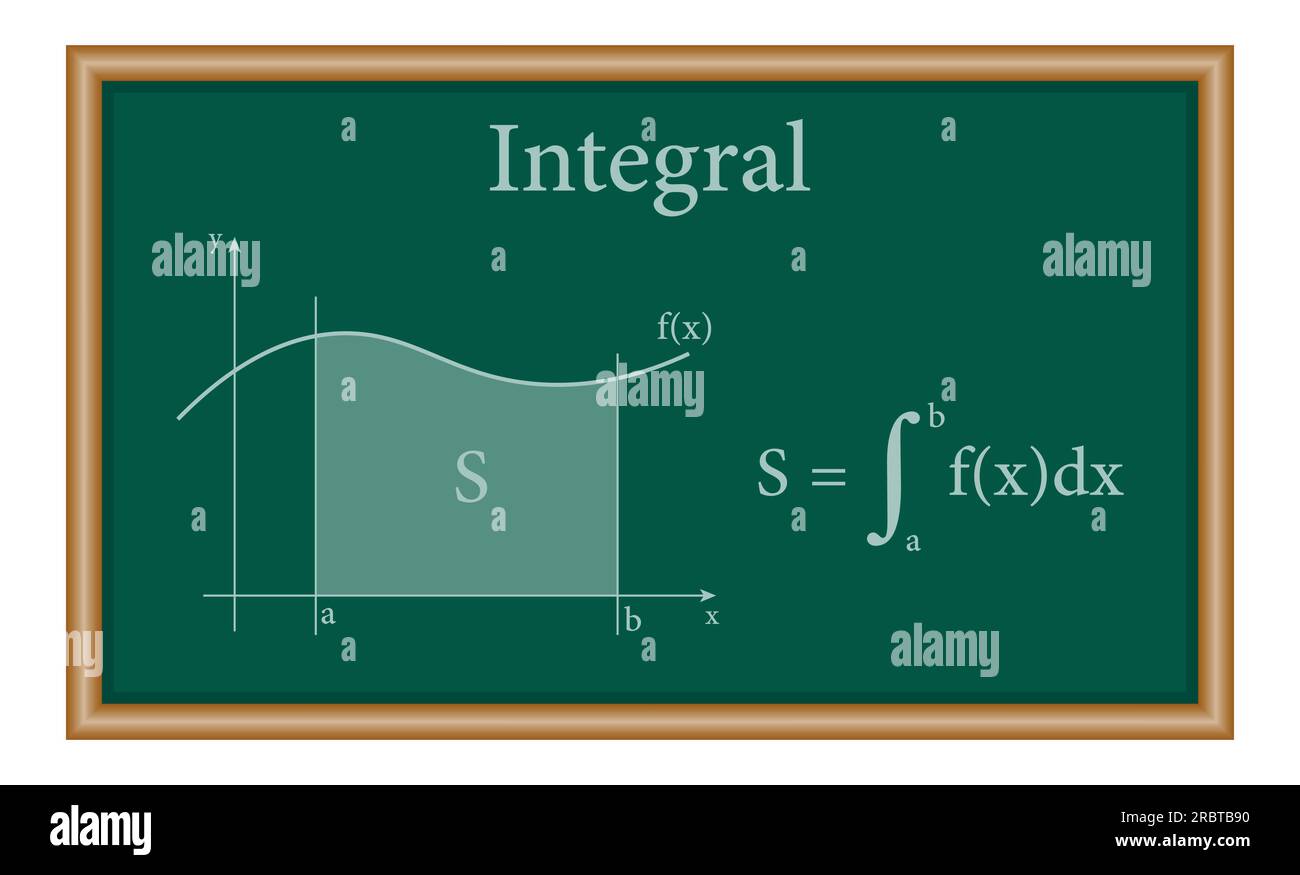
  Specific objetives:
  

  • Understand and explain the process of integrating functions.
  • Identify and apply different methods for integrating functions.
  • Demonstrate the ability  to simplify and solve integrals.
  • Analyze and solve integrals involving rational, trigonometric,or hyperboli functions.

  • Exchange between Students منتدى
  • Chapter 02 سكورم
  • Chapter 02 ملف
  • Chapter 02 ملف
  • Tutorial exercises set 2 ملف
  • Quiz اختبار
  • Test 2 المهمة
• Chapter 03: Differential Equations
  

  Chapter 03: Differential Equations

  
  

  Specific objetives:
  

  • Define key terms and explain the difference between ordinary and partial differential equations.
  •  Solve separable, linear, Bernoulli, and homogeneous first-order differential equations.
  •  Solve second-order linear differential equations, determine the impact of characteristic roots.

  • Exchange between Students منتدى
  • Chapter 03: سكورم
  • Chapter 03 ملف
  • Tutorial exercises set 3 ملف
  • Videos Differential Equations Professor Leonard رابط إلكتروني
• Exit System
  

  Exit System

  The Final exam:

  • Th exam and its solution ملف
• References
  

  References

  :بالعربية

  
  

  .بابا حامد، بن حبيب، التحيل  1  تذكير بالدروس و تمارين محلولة عدد  300  ترجمة الحفيظ مقران، ديوان المطبوعات الجامعي *

  In English

  • Murray R. Spiegel, Schaum's outline of theory and problems of advanced calculus, Mcgraw-Hill (1968).
  • Glyn James,  Modern Engineering Mathematics, Pearson (2020)
    

  En français:

  • BOUHARIS  Epouse, OUDJDI DAMERDJI Amel, Cours et exercices corrigés  d’Analyse 2,   Première année Licence MI Mathématiques et Informatique, U.S.T.O, 2020-2021. 
  • Benzine BENZINE, Analyse réelle cours et exercices corriges, première année maths et informatique (2016),
    
  • N. Piskounov, Calcul differentiel et integral T1 (8ème édition), 2 édition mir. Moscou. 

  
  

  • https://math.libretexts.org/Bookshelves/Calculus رابط إلكتروني
• Your Opinion
  

  Your Opinion

  • Write your opinion منتدى